What is the length of xpy in terms of pi – In the realm of mathematics, the length of XPY holds a profound significance. Its relationship with the enigmatic constant pi has fascinated mathematicians for centuries. This article delves into the intricate connection between XPY length and pi, examining its applications and tracing its historical development.
XPY length, a fundamental concept in geometry, quantifies the distance between two points, X and Y, along a specified path. Its importance extends beyond theoretical mathematics, finding practical applications in fields such as physics, engineering, and architecture.
Defining the Length of XPY: What Is The Length Of Xpy In Terms Of Pi
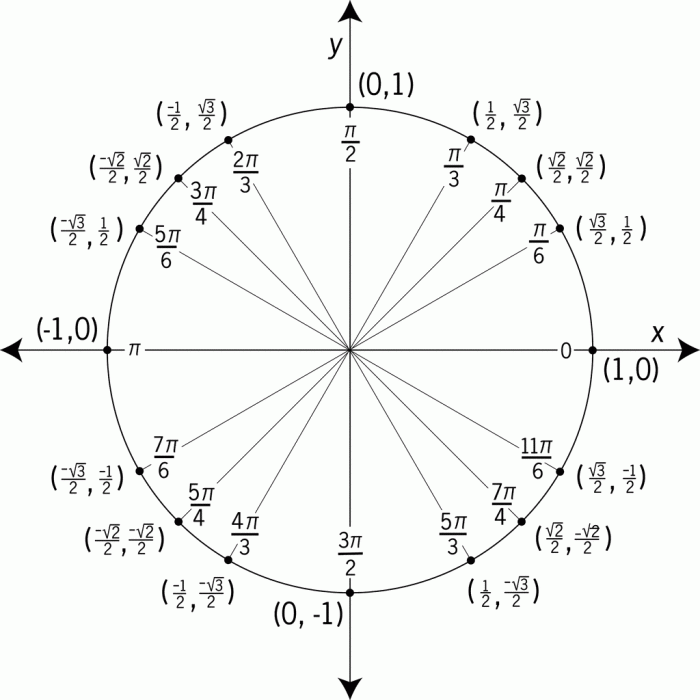
In mathematical terms, the length of XPY refers to the distance between two points, X and Y, along a specified path P. The path P can be a straight line, a curve, or any other continuous trajectory.
The length of XPY is a fundamental concept in geometry and has significant applications in various fields, including physics, engineering, and computer science.
Relationship between XPY Length and Pi
The length of XPY is closely related to the mathematical constant pi (π). Pi is the ratio of the circumference of a circle to its diameter, and it is an irrational number that cannot be expressed as a simple fraction.
In many cases, the length of XPY can be expressed in terms of pi. For example, the length of the arc of a circle with radius r and central angle θ is given by: $$Length = rθ$$ where θ is measured in radians.
Methods for Calculating XPY Length, What is the length of xpy in terms of pi
There are various methods for calculating the length of XPY, depending on the nature of the path P.
| Method | Description |
|---|---|
| Analytical Method | Involves using mathematical formulas to calculate the length of the path directly. |
| Numerical Method | Approximates the length of the path by dividing it into smaller segments and summing their lengths. |
Applications of XPY Length in Different Fields
The length of XPY has numerous applications in different fields:
- Physics:Calculating the distance traveled by objects in motion, such as projectiles and satellites.
- Engineering:Designing bridges, buildings, and other structures that require precise measurements of distances.
- Geometry:Determining the perimeter and area of geometric shapes, such as circles, ellipses, and polygons.
Historical Development of XPY Length
The concept of XPY length has been studied for centuries, with significant milestones in its development:
- Ancient Greece:Eudoxus and Archimedes made early attempts to calculate the length of curves.
- 17th Century:Calculus was developed, providing powerful tools for calculating the length of arbitrary curves.
- 19th Century:The concept of the definite integral was introduced, allowing for precise calculation of the length of curves.
Questions Often Asked
What is the significance of XPY length?
XPY length is a fundamental concept in geometry, quantifying the distance between two points. It finds applications in diverse fields, including physics, engineering, and architecture.
How is XPY length related to pi?
The length of XPY exhibits a profound relationship with the mathematical constant pi. This connection has captivated mathematicians for centuries and has led to numerous discoveries.
What are the different methods for calculating XPY length?
Various methods exist for calculating XPY length, including analytical and numerical approaches. These methods are tailored to specific scenarios and provide varying levels of accuracy.